Function Notation

Pronunciation: /ˈfʌŋk.ʃən noʊˈteɪ.ʃən/ Explain

Figure 1: Function notation diagram

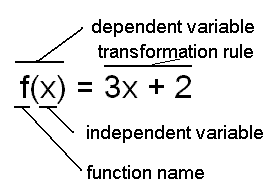
Function notation is a way to write functions that clarifies the name of the function, the independent variables, the dependent variables, and the transformation rule. In the example on the right, f(x) is the dependent variable, f is the function name, x is the independent variable, and 3x + 2 is the transformation rule.

References

1. McAdams, David E.. All Math Words Dictionary, function notation. 2nd Classroom edition 20150108-4799968. pg 83. Life is a Story Problem LLC. January 8, 2015. Buy the book
2. Bettinger, Alvin K. and Englund, John A.. Algebra and Trigonometry. pg 55. www.archive.org. International Textbook Company. January 1963. Last Accessed 7/11/2018. http://www.archive.org/stream/algebraandtrigon033520mbp#page/n72/mode/1up/search/notation. Buy the book
3. Goldrei, D.C.. Classic Set Theory: For Guided Independent Study. pg 4. Chapman & Hall Mathematics. July 1, 1996. Last Accessed 7/11/2018. Buy the book
4. Long, Edith and Brenke, W. C.. Algebra, First Course. pp 206-213. www.archive.org. The Century Co.. 1913. Last Accessed 7/11/2018. http://www.archive.org/stream/algebrafirstcour00longrich#page/212/mode/1up/search/notation. Buy the book
5. Ron Larson, Robert P. Hostetler. College Algebra. 7th edition. pp 191-192. Brooks Cole. January 5, 2006. Last Accessed 7/11/2018. Buy the book

Cite this article as:

McAdams, David E. Function Notation. 4/21/2019. All Math Words Encyclopedia. Life is a Story Problem LLC. https://www.allmathwords.org/en/f/functionnotation.html.

Image Credits

• All images and manipulatives are by David McAdams unless otherwise stated. All images by David McAdams are Copyright © Life is a Story Problem LLC and are licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.

Revision History

4/21/2019: Modified equations and expression to match the new format. (McAdams, David E.)
12/21/2018: Reviewed and corrected IPA pronunication. (McAdams, David E.)
7/9/2018: Removed broken links, updated license, implemented new markup, implemented new Geogebra protocol. (McAdams, David E.)
2/5/2010: Added "References". (McAdams, David E.)
8/2/2008: Added transformation rule. (McAdams, David E.)
7/1/2008: Initial version. (McAdams, David E.)